Crack the Shell of Nut Draw Strategy

Nut draws hold obvious appeal as betting and raising candidates. They benefit from fold equity, sometimes even making better hands fold, while also having the potential to win big pots when opponents do not fold immediately.

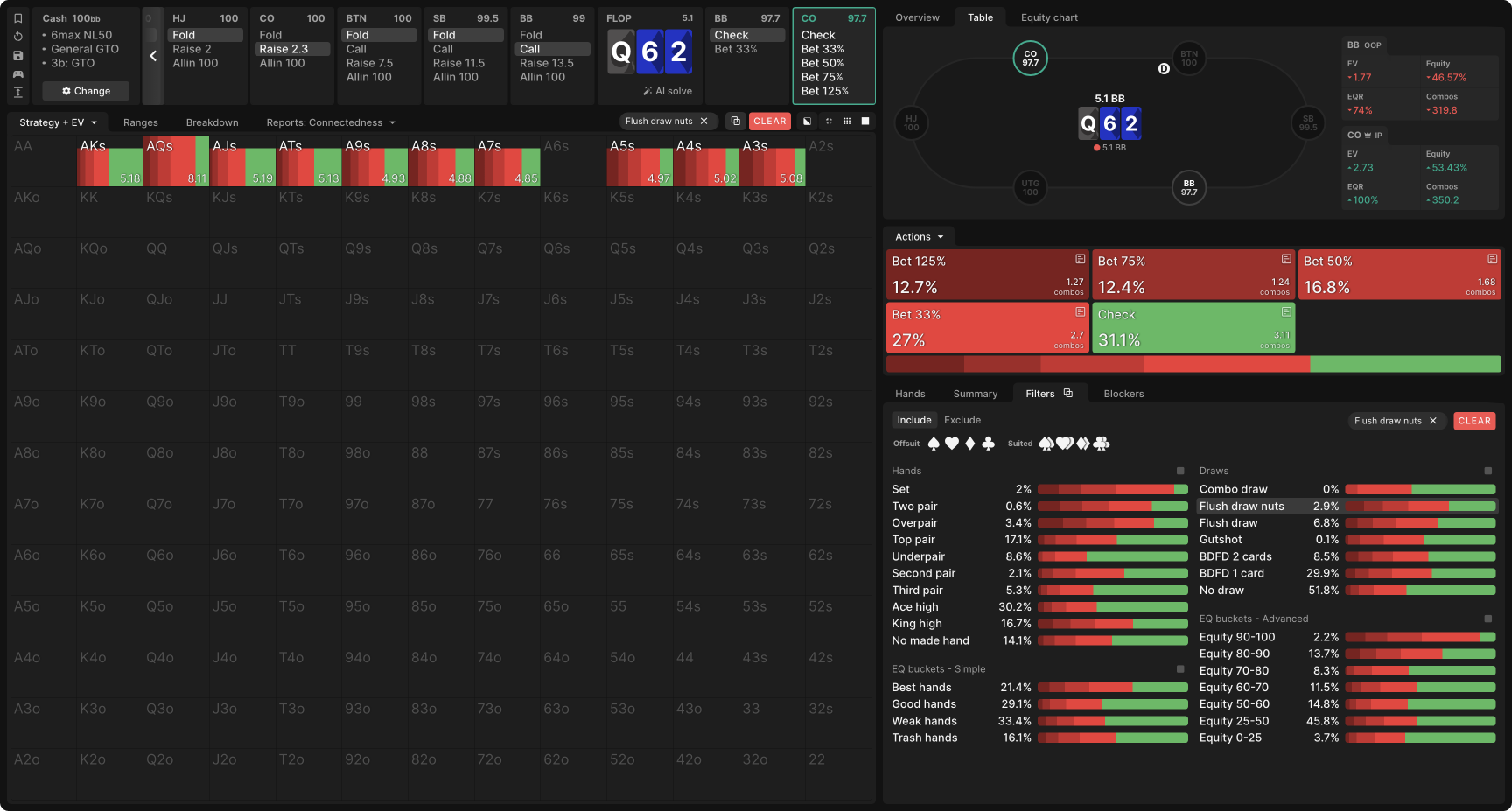
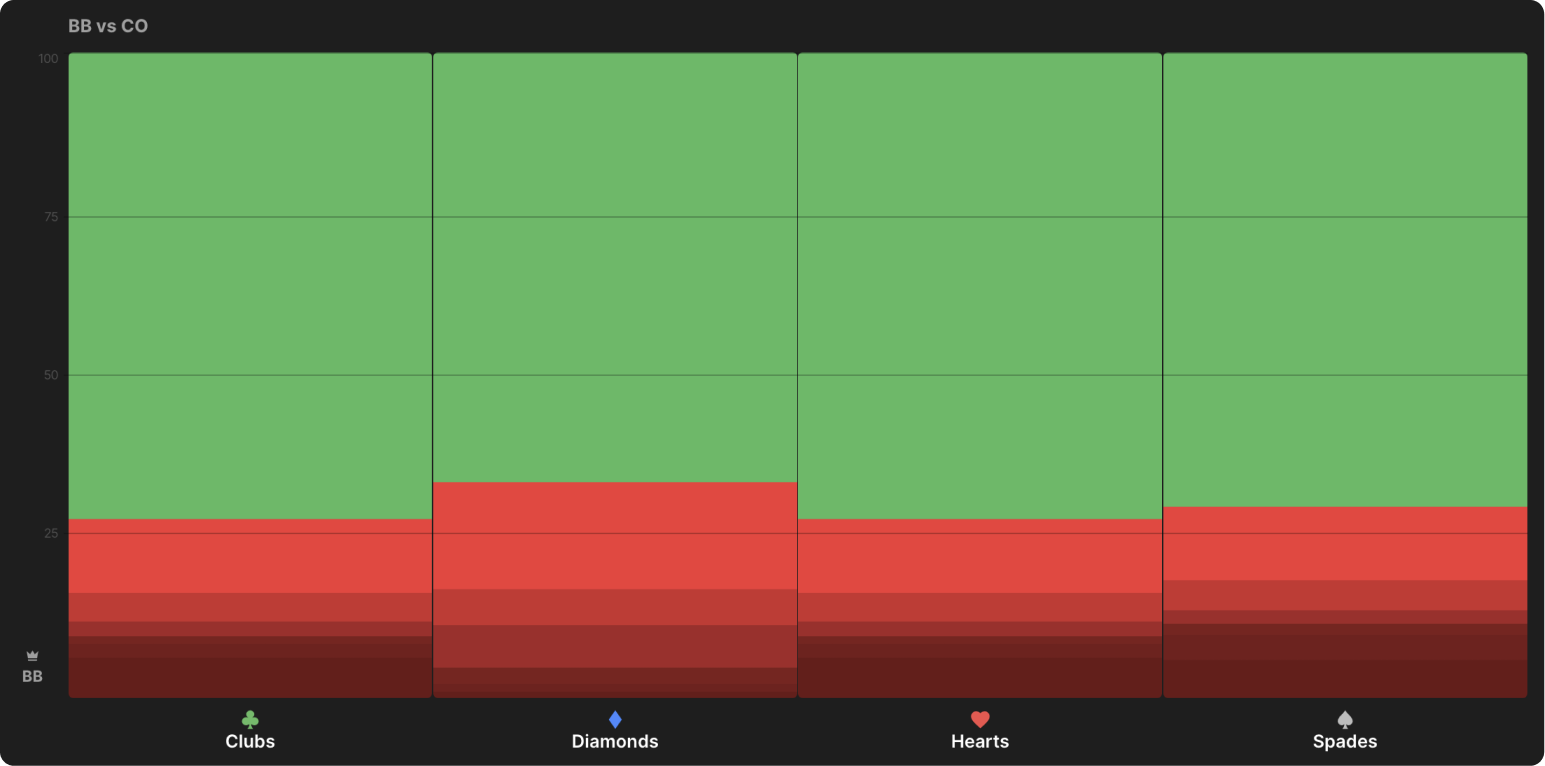
Yet solvers do not consistently play nut draws aggressively. For example, in a 100bb CO vs BB single-raised pot (SRP), CO continuation bets their nut flush draws just 69% of the time on a Q♠6♦2♦ flop:

Despite the obvious appeal of c-betting nut flush draws, solvers do not consistently always bet them.

CO’s overall c-betting frequency—as in the frequency of their whole range, not just of their nut flush draws—here is just 49%, so they are playing nut flush draws more aggressively (69%) than their average hand. Yet none of them are pure bets, and when they do bet, they all mix across all four bet sizes.

What’s Going on Here?

As I put it in the blog post: “it’s good to be capable of making a flush on the turn when you check back the flop.”

There’s a broader principle of poker strategy at work here: it’s dangerous to cap your range. An opponent who can easily have the nuts themselves and can correctly predict you will rarely have nutted hands can bet big with a polar range, turning even your strongest hands into mere bluff catchers. The deeper the effective stack, the more dangerous this is.

This is not an ironclad rule. You do not need to warp your flop strategy around the risk of the bottom card pairing on the turn and river and you not having any quads in your range. But when it comes to common scenarios like a third flush card turning, solvers typically prepare by scattering their nut draws across all their actions.

Of course, solvers don’t think of it as planning ahead. They don’t “think” at all (as far as we know). They maximize EV by responding to incentives and addressing potential leaks that could be exploited. If a solver is mixing a hand across multiple actions, that hand must necessarily have the same equilibrium EV for all those actions. This means that if you were to bet all your nut flush draws in this scenario, your opponent could exploit that in some way, presumably with the aforementioned big bets on branches of the game tree where you can’t have the nuts. That’s what incentivizes you to show up with the nuts on those branches every now and again.

Let’s Experiment!

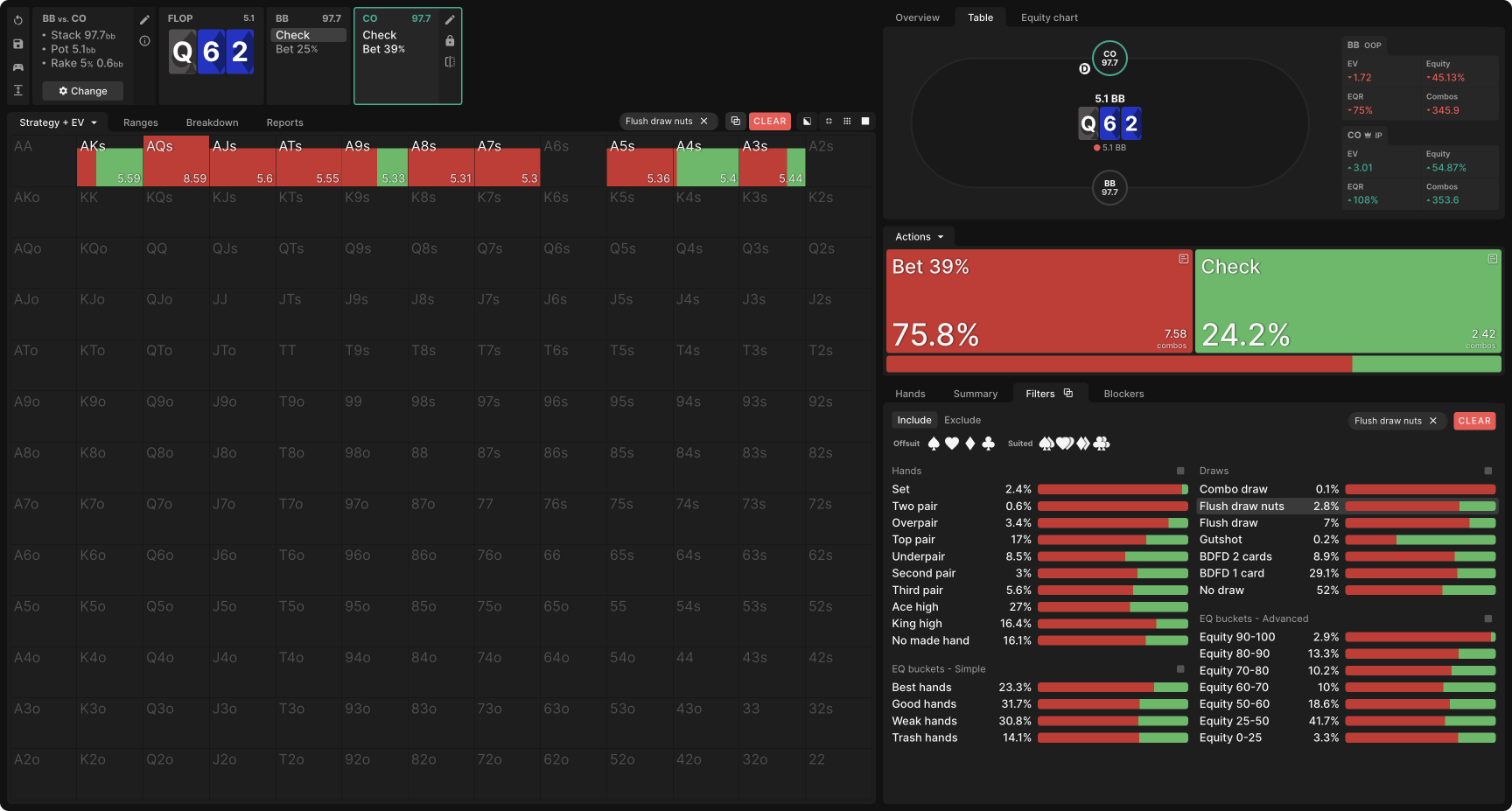
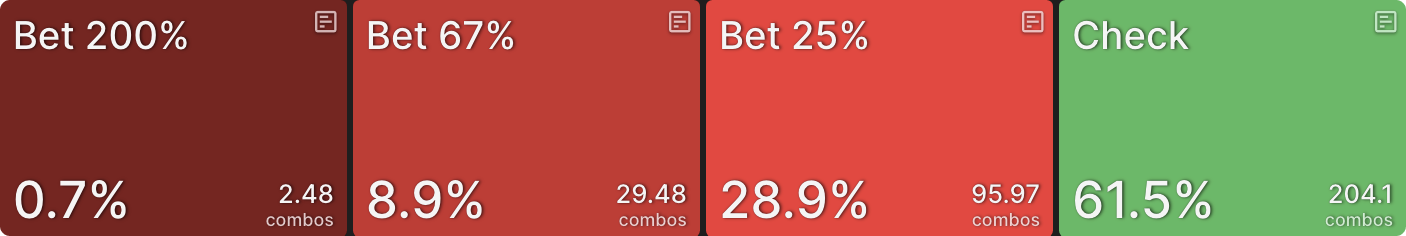
We can test and explore this idea using nodelocking. To do that, we’ll need a Custom solution. While we’re at it, let’s also simplify the decision tree, since we’re more concerned with whether to bet than with how much to bet. When only a single bet size is permitted, CO c-bets 70% of their range on Q62tt but 76% of their nut flush draws, demonstrating once again that such draws are indeed better-than-average betting candidates:

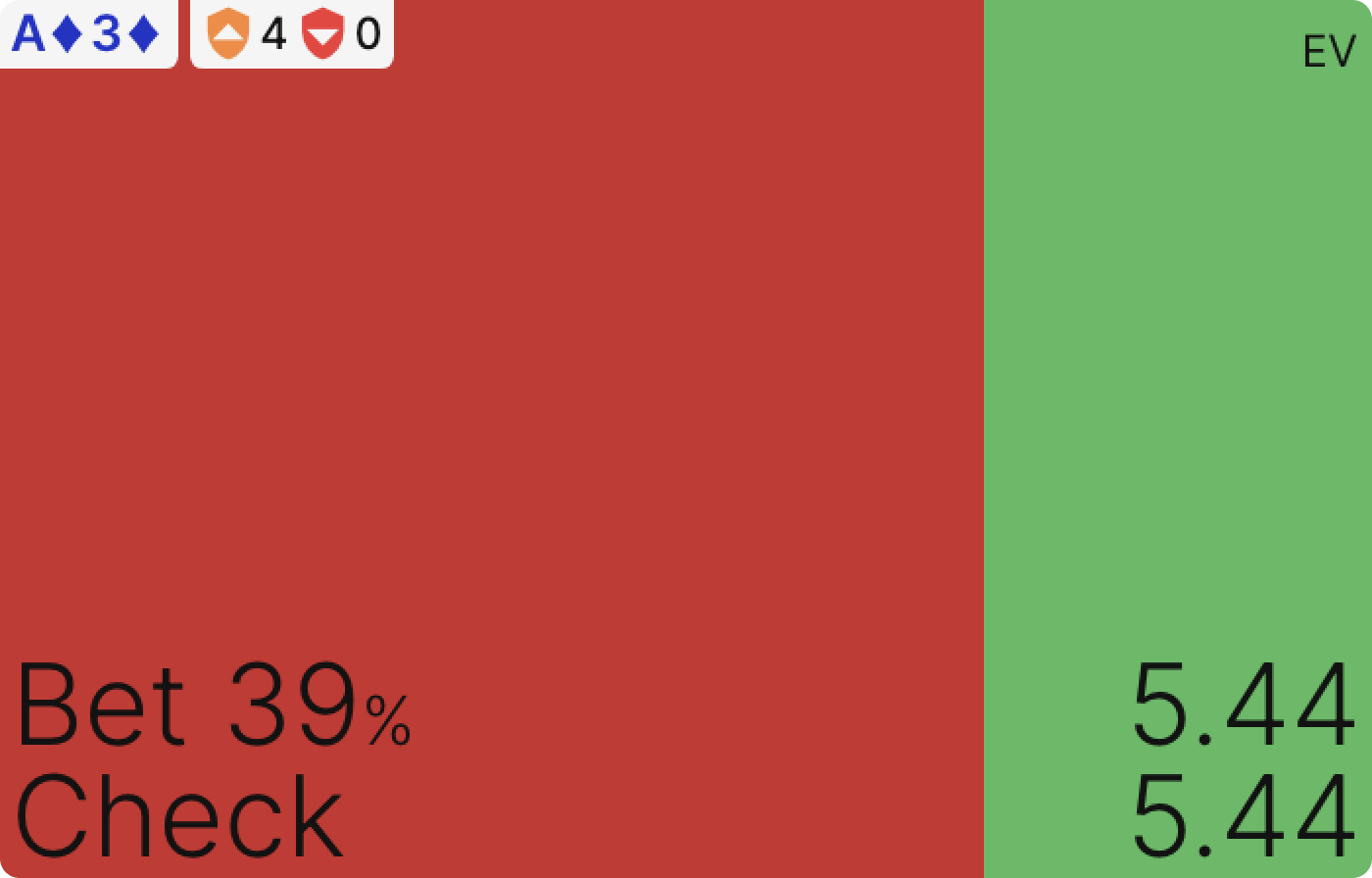
The solver does now show a preference for betting some combos over others, though not a strong one. A♦8♦, for instance, appears to perform 6bb/100 better as a pure bet. A♦3♦ has the same EV as a bet or check, so it plays as a mixed strategy.

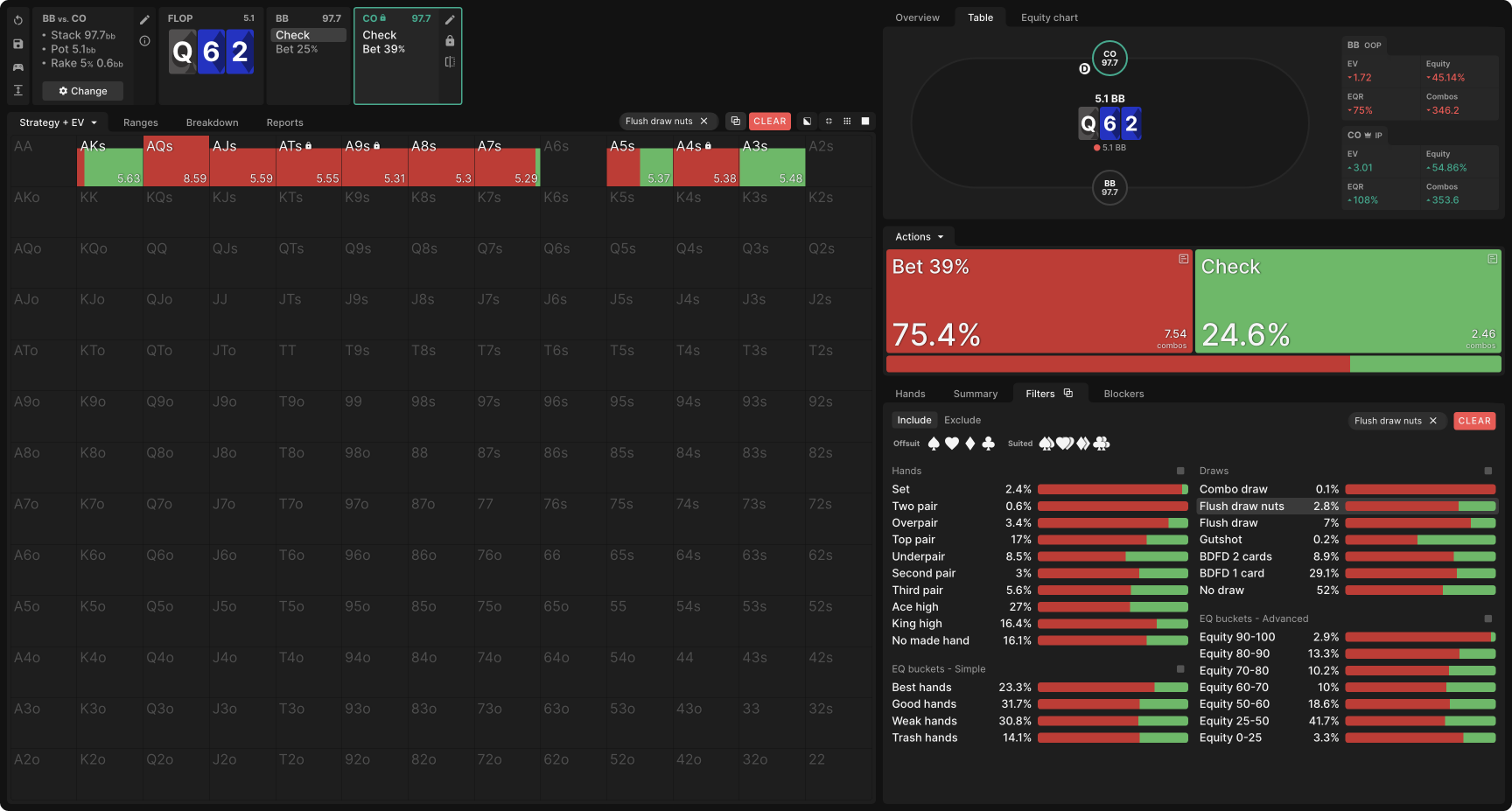
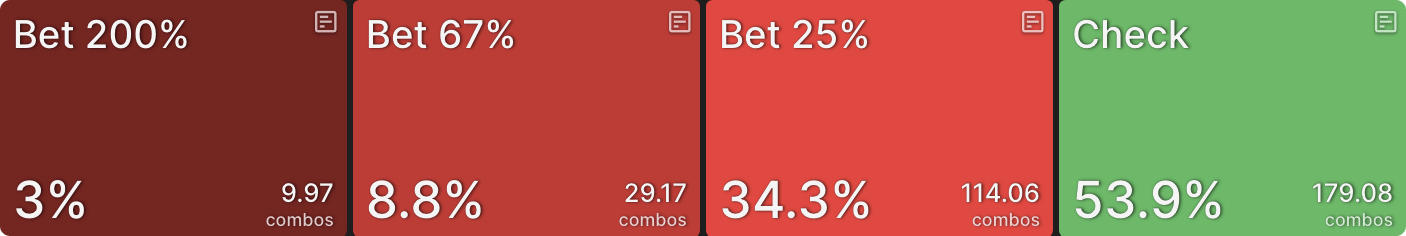
A♦9♦ is one of the nut flush draw combos that mixes in a decent chunk of checking at equilibrium. But if we lock CO’s strategy to pure bet A♦9♦, they compensate with more checking from their other mixed strategy combos: AK, AT, A4, and A3.

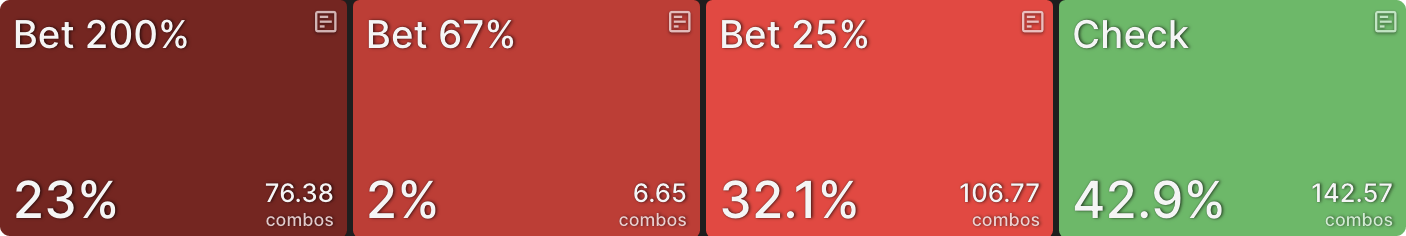
If we lock A♦9♦, A♦T♦, and A♦4♦ as pure bets, then A♦7♦ and A♦5♦, which were pure bets at equilibrium, start mixing some checks to compensate:

Notice how CO’s checking frequency remains relatively stable even as we introduce more fixed rules to their betting strategy. In fact, even their EV remains consistent despite being forced to play more and more combos “suboptimally.”

Maintaining the right frequency of nut draws on each branch of the game tree is more important than taking a certain action with a certain combo.

There are reasons why the solver prefers betting some combos, but with the exception of A♦Q♦, which is also top pair, they are not terribly important reasons. One combo may unblock a few more of BB’s folds or have slightly better pair outs. When armed with a dual-core processor’s computing power, CO might as well use those minor differences as tiebreakers when determining which combos to bet and which to check. But they are merely tiebreakers, and the method by which you choose which combos to bet is not terribly important.

The main takeaway here is that CO’s equilibrium strategy will include some nut flush draws in both their betting and checking ranges, and maintaining the right frequency of nut draws in each is more important than taking a certain action with a certain combo. Computers can make exceedingly precise distinctions, but as a human, you can choose heuristically—betting higher kickers and checking lower ones, for instance—or even arbitrarily, by checking 25% of the time with all combos.

A Note on Using Filters

This principle applies to any flop where CO has a significant checking range. I chose Q62 specifically, however, because none of CO’s nut flush draws are combo draws on this flop. On a flop like QT2, the “Flush draw nuts” filter would not include A♦K♦ and A♦J♦. Adding the Combo Draw filter would capture them, but it would also capture K♦J♦ and other non-nut combo draws. You must be careful, when using filters, to capture only and exactly the combos you’re interested in.

Playing Flush-Completing (Diamond) Turns

When Flop Checked Through

The following report shows BB’s betting strategy across all turns after CO checks back the flop. Notice that diamond turn cards are bet least often, at equilibrium, for a big sizing:

We can’t run reports on Custom solutions, but we can pick an otherwise innocuous diamond like the 8♦ and compare strategies to see what changes due to CO betting more flush draws on the flop.

We’ll also want to change the parameters of our Custom solution a bit, since our hypothesis is that overbetting will be a more important part of BB’s strategy when CO caps their range. Instead of determining all bet sizes automatically, I’ve given both players Fixed betting options of 25%, 67%, or 200% pot on the turn and river.

BB’s equilibrium strategy does not entail much overbetting on a 8♦ turn:

When CO is locked to bet all their nut flush draws on the flop, big bets on this turn become more appealing after CO does not bet:

Keep in mind that CO still has flushes in this scenario, as they compensate for betting all their nut flush draws by checking more low flush draws. So BB can exploit by overbetting even though CO has a fair number of flushes in their range.

When CO is forced to bet all their flush draws on the flop, BB can really put the screws to them on flush-completing turns after CO checks the flop:

Conclusion

Pure betting your flush draws on the flop is not necessarily bad. Indeed, against opponents who do not check-raise aggressively, it may be correct to c-bet your entire range, even in spots where the equilibrium strategy involves a lot of checking. But it is a choice, and you should approach it as such, with a clear-eyed understanding of the risks and rewards.

How could your opponents exploit your choice? Do you think they will actually act on those exploits? Will they perhaps even lean too far into the exploits, incentivizing you to lean the other way and counter-exploit them as I did back in 2014?

The better you understand equilibrium solutions—not just what they look like but why they look the way they do—the better equipped you will be to identify the options on the table and choose the one that will perform best against a given opponent.